Fig. 1.2. Graphical representation of the discrete strategy of buyer’s and seller’s market behavior represented by the two points, A(p^D, q^D) and B(p^S, q^S), in the two-dimensional price-quantity space at some particular moment in time for the model grain market. p^D = 280,0 $/ton, q^D = 50,0 ton/year, p^S = 285,0 $/ton, q^S = 52,0 ton/year.

We will speak (for the sake of brevity) of this aggregate agents’ movement as market behavior, and sometimes as the economy evolution over time. All these terms are essentially synonymous in this context of discussion. Thus, by putting up desired prices and quantities as their quotations, buyers and sellers take part in the market process, proceeding here in the format of negotiations between bargaining people (homo negotians) seeking to bargain for the best terms for themselves in concluding a deal and achieving market goals. Let us note that in reality the actions of market agents include the procedures of concluding final deals along with quotations, but these procedures are automatically accounted by means of changing quotations by market agents after the conclusion of deals. Therefore, there is no need to Explicitly include the procedure of concluding transactions in the structure of agents' actions, it is enough to take into account only the quotation process in the course of trading.

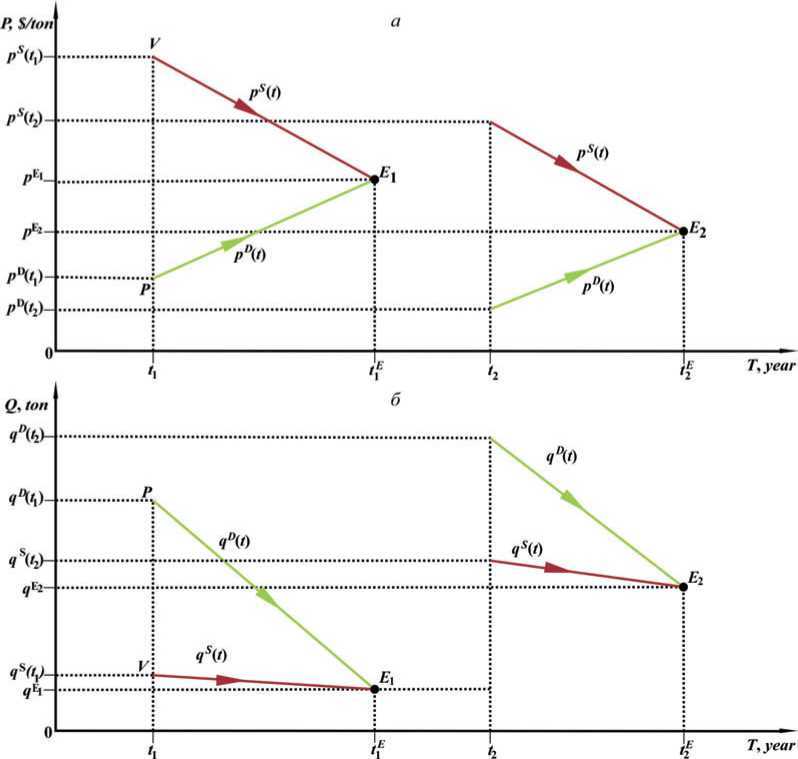
Fig. 1.3. Diagram of buyer and seller trajectories. The dynamics of the classical two-agent market economy in the economic space of price (a) and quantity (b) is depicted. Together, both parts of the figure represent the evolution of the economy over time in two-dimensional PQ-space.

This whole trading process, or simply bargaining, can be interpreted as a dynamic business exchange game between buyer and seller with the purpose of making a profit or achieving some other goal.

Let’s suppose that the negotiations went well and ended with the conclusion of a sale and purchase deal at time t₁^E. This means that at that moment in time the values of prices (p^D(t) and (p^S(t)) and quantities (q^D(t) and (q^S(t)) in the quotations become equal, since obviously only specific mutually agreed price р₁^Е and quantity q₁^Е of goods can be specified in the contract. Let us assume that in this bargaining model it makes some sense to call these price and quantity values the market price and quantity of the commodity and to assume that the market itself comes to or reaches its equilibrium state at these price and quantity values. Formally, this is described using the following equations for the market price and quantity:

So, for the two-agent model we obtained this trivial but significant result: the very fact of reaching equilibrium makes it possible to conduct a transaction and maximize the volume of trades in monetary terms. In this simple case the conclusion is quite obvious: no agreement, no equilibrium, no transaction, trading volume is zero. But we will further show that this conclusion has a rather universal nature, which agrees with the postulated trade volume maximization principle. By the way, it is easy to show that in the framework of the neoclassical theory the maximum trade volume in natural terms, i.e. the maximum quantity of traded goods is reached at the equilibrium point.

Further on, since life does not stand still, the buyer and the seller can meet again and make new deals, but under new conditions and, obviously, with other prices and quantities, then for convenience we will call р₁^Е the first market price, and q₁^Е the first market quantity. Thus, at time t₁^E, the interests of the buyer and the seller have coincided for the first time, and they have been optimally satisfied by concluding a sale-purchase transaction. In this case agents, naturally, in the course of the market process (negotiations and changes in quotations) implicitly took into account the influence of the external environment and institutional factors on this and other markets, i.e. the economy as a whole. Here one can notice a similarity in the motion of the economic system in economic space, described by the trajectories of the buyer р^D(t) and q^D(t) and the seller р^S(t) and q^S(t), and in the motion of the two-particle physical system in real space, described by the trajectories of particles x₁(t) и x₂(t), which, by the way, are also the result of a certain physical maximization principle, namely, the principle of least action on the physical system.

The noted analogy with the physical system suggests using a similar mathematical body, analytical and graphical. In Fig. 1.3, to begin with, we provided a graphical representation of these trajectories of agents' motion as a time function using suitable coordinate systems time-price (T, P) and time-quantity (T, Q), similar to the construction of particle trajectories in classical mechanics. Please, note that figure 1.3 reflects a certain standard situation in the market, when buyer and seller intentionally meet at a point in time and start discussing a potential deal by mutual exchange of information about their conditions, first of all, desired prices and quantities of goods. During the negotiation, they continuously change their quotations until they agree to final terms on price р₁^Е and quantity q₁^Е at the time t₁^E. Such a simple «negotiation» market model is applicable, for example, to the economy of a fictional island on which, let us say for certainty, once a year there is a negotiated grain trade between a farmer and a hunter. To introduce some certainty, let us assume that they use the American dollar, $, for settlement. For clarity, in Fig. 1.3, as in the following figures, we use arrows to reflect the direction of agents’ movement during the market process.

So, in our classical negotiation model, up to the moment t₁ the market is in the simplest dormant state, there is no trade at all. At time t₁, a buyer and a seller of grain appear in the market, and set their initial desired prices and quantity of grain: р^D(t₁), р^S(t₁) and q^D(t₁), q^S(t₁) points P and V in the graph show the position of the buyer and the seller at the initial moment of time t₁ when trade negotiations begin. Naturally, the desires of buyer and seller do not immediately coincide; the buyer wants a low price, but the seller is fighting for a higher price. However, both need to reach an understanding and subsequent deal, otherwise the farmer and the hunter will have a difficult next year. The negotiation process continues, with the market agents' process of changing their quotations reflecting its progress. As a result, the positions of the market agents converge, and they coincide at time t₁^E, which corresponds to the point of trajectories intersection Е₁ on the graphs.